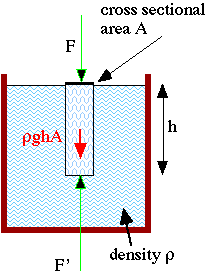
 Look at
the little cylinder of fluid with cross sectional area A and height
h. We know that it has a mass
Look at
the little cylinder of fluid with cross sectional area A and height
h. We know that it has a massWhen you dive in your local pool or the ocean, your ears hurt more and more as you dive deeper and deeper. We understand that this is due to the water pressure that increases with increasing depth. Here we will derive how the pressure depends on depth:
 Look at
the little cylinder of fluid with cross sectional area A and height
h. We know that it has a mass
Look at
the little cylinder of fluid with cross sectional area A and height
h. We know that it has a mass
where
![]() g
is the force of gravity, pointing downward with magnitude:
g
is the force of gravity, pointing downward with magnitude:
The force up on the bottom is F' and down on the top is F. Since the body is symmetric, the sideways forces must cancel. So we get from the above force equation:
But from the definition of pressure, we know that:
where p0 is the pressure at the surface and p is the pressure at a depth h. Putting this in, we obtain:
This is the pressure-depth relation.
Please note:
To arrive at this result, we have assumed that the density,$\rho$ , does not change as a function of the depth. This is a consequence of the basically incompressible nature of the liquid. For gases, the above formula holds only approximately.
© MultiMedia Physics, 1999