Centripetal Acceleration

The word "centripetal" means "center-seeking" which is pretty descriptive in the case of centripetal acceleration. In uniform circular motion the speed does not change, but the direction of the velocity vector continuously changes. As you can see from the animation below, the direction of this vector is always tangential to the circle, and thus it is continuously deflected towards the center. The acceleration causing this deflection is thus called centripetal acceleration.

_c = - a_{c r}

where _r is a unit vector in direction of the r-vector,

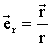
and the magnitude of the centripetal acceleration is