 Potential
due to a single charge:
Potential
due to a single charge:Since we already calculated a few electrostatic potential energies, we can calculate the electrostatic potential for these cases as well.
 Potential
due to a single charge:
Potential
due to a single charge:
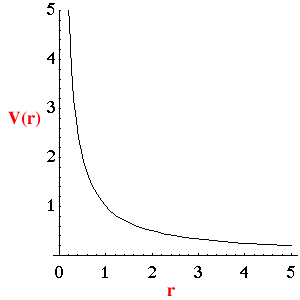
From the discussion of the last section,
and the definition of V, for a charge Q at the origin, in a medium of
permittivity ![]() is :
is :
![]()
This potential is also known as the
Coulomb potential.
 Potential
in a constant electrostatic field:
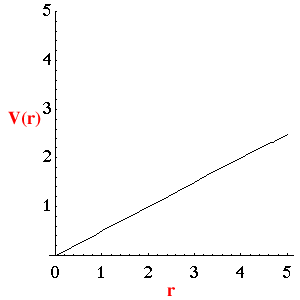
Potential
in a constant electrostatic field:
Potential difference in a constant electric field: VAB
= - E
Therefore potential in a constant field is :
Potential at a point due to a number of charges:
The potential due to a single charge is a scalar. If we have a number of charges, the net potential at any point due to the entire charge distribution is found by simply adding the contribution of each charge, individually.
The potential at the point ![]() is due to one of the charges is unaffected by the presence of the
other charges. That is why we can calculate the contribution at
is due to one of the charges is unaffected by the presence of the
other charges. That is why we can calculate the contribution at
![]() due to
each charge separately, and simply make the algebraic sum. This is
called the Principle of
Superposition.
due to
each charge separately, and simply make the algebraic sum. This is
called the Principle of
Superposition.
© MultiMedia Physics 2000